Quantum Computation is a field of immense potential. From drug manufacturing to route-optimization- from AI to cryptography, scientists are dreaming of solving problems which can easily be considered intractable classically. Among all these aspirations and hopes, is it too far fetched to imagine classical computers becoming obsolete one day and quantum computers taking over? Adopting two different approaches can help us answer ourselves. Firstly, let us find out whether quantum computers can, in theory, do all that classical ones can.
Can Quantum Computers Replace Classical Computers?
Theoretically, yes.
According to the correspondence principle of quantum mechanics (by Niels Bohr in 1920), under suitable conditions, quantum systems imitate classical systems following the intuition that all macro-classical systems are actually quantum systems when observed at the micro-level. So, all classical systems can be simulated using a quantum machine just as we observe quantum systems as classical (on the macro-level) in disguise.
In Computer-science terms, all classical circuits can be implemented via quantum circuits. Let every classical computation be a boolean function as follows:
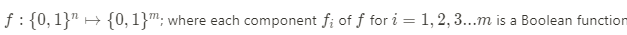
with truth tables. Now based on the truth tables, we can create classical circuits using AND, NOT and OR gate. Again, these gates can be implemented with a combination of NAND gates in classical circuits, hence NAND gate is universal for classical computers.
However, NAND gates are not reversible which is a must for Quantum circuits. To make classical circuits reversible, we use Toffoli gates instead of NAND as Toffoli gates are reversible. Now, the toffoli gate can be easily replaced by the quantum equivalent of classical toffoli gate: Quantum Toffoli Gate and in this case, we have to pass on inputs in the form of quantum states rather than classical bits. Instead of strings of bits, computation will be carried out on quantum bit-string: for example,
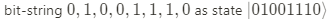
without directly relying on superposition.
From the perspective of Quantum Complexity theory: if P resembles problems that are easily solvable by classical computers and BQP resembles those by quantum computers,
which agrees with what we have just deduced.
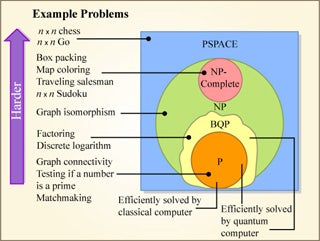
Voila! We now have, at least in principle, a classical equivalent quantum machine that can compute any classical circuit with a quantum circuit. Does that mean classical machines will slowly become redundant with gradual progress of quantum devices?
“Will” quantum computers replace traditional computers?
Back to reality, very unlikely.
Although in theory, classical computers can be replaced by their quantum counterparts, the question is why would we do so. There are some obvious reasons behind why this might not happen, submitting to limitations of quantum machines.
Limitations:
- Nature of Qubits: For classical computers, a great effort is put into ensuring chips of bits are not interacting with each other. Quantum computers, on the other hand, take advantage of inter-qubit interaction. In fact, this connectivity is something they leverage to gain advantage over classical computers. However, this interactivity often causes the states of the qubit to change erroneously, which is detrimental to calculation.
- More qubits, more error: When computing classically, using N bits, we can compute maximum up to N number of calculations at once; whereas, in Quantum machines, using N number of qubits will allow us to manage 2^N number of calculations at once. However, the addition of qubits comes at a cost. Quantum computers with more qubits mean lesser connectivity. Lesser connectivity among qubits implies the necessity of more quantum gates for simple calculations. And gates are very prone to errors. Manipulating qubits is a real challenge and this might result in alteration of the expected outcome. Today’s quantum devices are nowhere near to a coherently connected qubit model.
- Qubits are incredibly tough to maintain: Qubits are highly sensitive- they respond to slightest change in environment and lose the data encoded in the form of states. Take super-conducting qubits, for example, which need to be stored in a dilution refrigerator, making them unlikely to be found in place of your living-room PC. Again, all this sensitivity works against decoherence time. Quantum memory is now about some micro-seconds; whereas to solve real-life problems, it needs to be stretched to minutes, if not hours.
- Range vs direct answers: This is an inherent property of quantum computers that they provide a range of solutions with estimated probabilities to a problem instead of one specific answer. Oftentimes in reality, the calculations that we carry out are not as complex and not dependent on too many parameters that may result in a range of plausible outcomes. Simplicity comes to play in case of classical machines as they provide the only answer with direct inference. So we will be ultimately dependent on classical computers to re-check the outputs with high possibilities.
- Error mitigation is hard: Error is a disposition, and it is almost impossible to make error free quantum computers. A lot of talent and resources are being poured into coming up with a quantum machine that can detect its own error and rectify itself. Currently we are in the NISQ-era of quantum machines where we try to mitigate errors instead of correcting them and thus cannot attempt to compute complex algorithms (like Shor’s algorithm) which requires close-to-ideal quantum devices.
- Algorithmic Disadvantage: The classical world of algorithms is being developed for many years now and it is obvious that quantum is at baby-steps. There are a lot of classical algorithms that are developed only to solve certain problems and they do that better than general algorithms of quantum machines in theory. To catch up with the current pace of classical development is again quite not realistic considering that quantum computation is not an up-gradation, rather a completely new way of doing so. Insights are not always clear and sometimes, it is really hard to grasp the intuition.
What can we expect, instead?
Perhaps quantum devices were never meant to replace classical ones. The world of computation can be rewired to work within a symbiotic mutualism of quantum-classical computers. Here, the part of algorithm which can be effectively accomplished without leveraging quantum properties is done classically. Only when properties of quantum mechanics like: superposition, interference and entanglement can result in a boost of computation, quantum machines are used. For instance, in Shor’s algorithm quantum device does what cannot be efficiently done using a classical device: estimate the phase of an eigenvector of a unitary operator and the rest is carried out classically. Similar approach is found in the case of VQE: the quantum part simulates the molecule and a classical optimizer tweaks the parameters. This particular model namely, Quantum-Classical Hybrid Model can accelerate the use of quantum devices to solve real-life problems.
Fields such as AI, drug manufacturing, chemistry simulation, transportation are expected to be hugely benefited from such a model. Again, quantum cryptography is an emerging field that relies on Shor’s Algorithm with a view to breaking the current encryption system based on prime factorization. Although machines on your desk would not be replaced anytime soon, we can certainly see a future when on cloud large computations, to solve real life problems, are being carried out on quantum devices, accessed via classical machines as well a revolution in quantum-safe information encryption.